Mathematics Of Poker Review
Essential Poker Math. Alton Hardin, who has been playing poker for over ten years and is the founder and creator of the MicroGrinder Poker School have written this excellent book. This piece will teach you elementary poker math and how to use it to increase your winnings. It will cover probabilities and odds, expected value, pot equity, ratios. Amazon.in - Buy Poker Math That Matters: Simplifying the Secrets of No-Limit Hold'em book online at best prices in India on Amazon.in. Read Poker Math That Matters: Simplifying the Secrets of No-Limit Hold'em book reviews & author details and more at Amazon.in. Free delivery on qualified orders.

Mathematics Of Poker Review 2020
The Mathematics of Poker
by Bill Chen and Jerrod Ankenman
Diligent readers who invest the necessary effort to follow Chen and Ankenman’s arguments and consider their implications, however, will not be disappointed. Careful study of this text will reveal a world of insight into poker concepts such as value betting, range balancing, and optimal strategy. Although more could have been done to elucidate its practical applications, The Mathematics of Poker is nevertheless an extremely valuable text for any poker player willing to give it the thoughtfulness it deserves.

It is not an easy read, but it should not be beyond the grasp of anyone with a high school education. Game theory is serious mathematics, and nearly every page of this book is packed with equations, charts, and graphs. This looks intimidating, but in fact, the authors do all the heavy lifting and help a diverse audience follow along in a variety of ways. For the real mathematicians, they show their work and occasionally suggest follow-up problems that readers might consider attacking on their own. However, they bracket these sections so that the mathematically challenged can skip past them to the (relatively) plain-language explanations of the process and results that follow. Chen and Ankenman do a remarkably good job of elucidating the conceptual meaning of equations and solutions, and every chapter concludes with a summary of the “Key Concepts.” Still, a passing acquaintance with statistical notation, graphical representation, and high school algebra is all but required to make sense of the text.
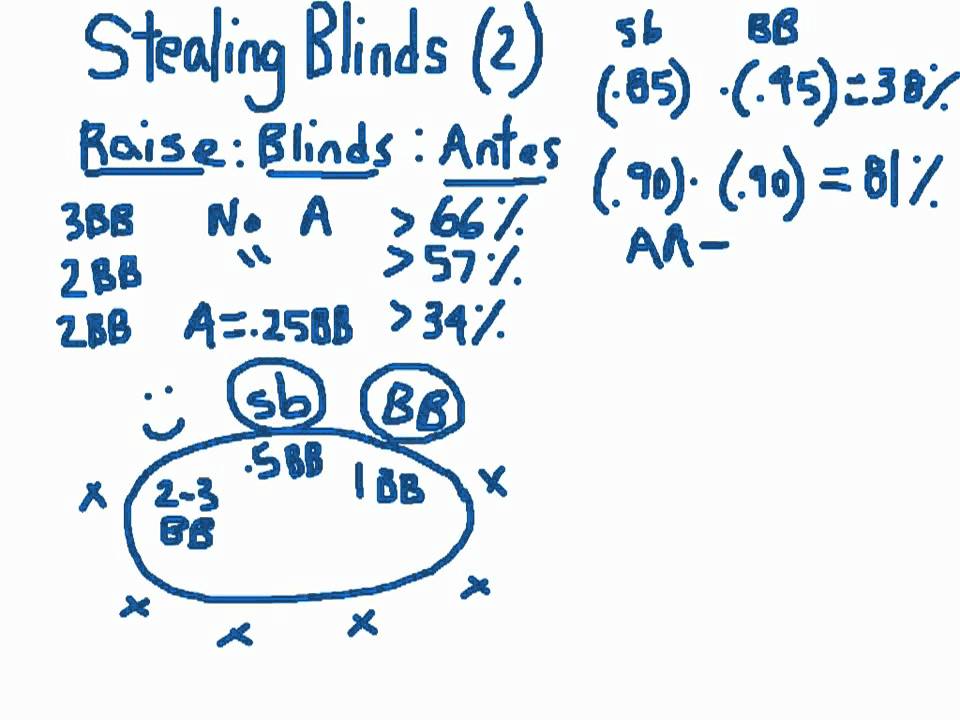
Mathematics Of Poker Review 2
After some opening chapters that cover concepts like variance, sample sizes, hand reading, and pot odds, Chen and Ankenman introduce the concept of optimal strategy, a style of play that cannot be exploited even if your opponents knew ahead of time exactly how you would be playing. In other words, they are interested in finding solutions such as the exact ratio of bluffs to value bets that would make your opponent indifferent to calling or folding with a weak made hand on the river.
All commonly played versions of poker are far too complex to solve with the tools of game theory, however. Instead, poker must be attacked indirectly, through a series of “toy games” that represent highly simplified poker situations. One oft-revisited example involves two players each dealt one card from a three-card deck containing exactly one A, one K, and one Q. Throughout the book, the authors consider situations where the second player to act knows what card his opponent holds, situations where he does not have this information, situations where the first player is forced to check dark, and finally a full-street game where neither player knows the other’s card but may bet, check, or raise as he sees fit.
The text is very helpful in explaining the optimal strategy for each player in each game and how the addition of new strategic options affects these results. Still, the ultimate solution is nothing but the optimal strategy for a game that no one will ever play. Undoubtedly, understanding what optimal play entails and how it is derived can be enormously valuable at the poker table. But these games are accompanied by a few sentences, at best, explaining their relevance to actual poker situations.
Part of my frustration stems from the fact that the tidbits of practical advice that Chen and Ankenman do include are tantalizingly thought-provoking: “Bluffing in optimal poker play is often not a profitable play in and of itself. Instead, the combination of bluffing and value betting is designed to ensure the optimal strategy gains value no matter how the opponent responds”; “Betting preemptively is a perfect example of a play that has negative expectation, but the expectation of the play is less negative than the alternative of checking”; “it’s frankly terrible to find oneself in a situation with a marked open draw.” Any of these insights is worthy of several pages of extrapolation, but this work is largely left to the reader.
This is more than an error of omission. When Chen and Ankenman do directly address the question of how their material translates into poker strategy, they concern themselves overly much with pursuing optimal versus exploitive strategies, which is to say strategies that deviate from unexploitable, or optimal, play in order to capitalize on perceived weaknesses in an opponent’s strategy.
Their stated reasons for this preference are that it is difficult to determine another player’s strategy with certainty and that opponents will eventually change their play to counter your exploitation. While these are both reasonable concerns, neither is prohibitive. Although certainty is impossible, the ability to make quick and reasonably accurate assessments of a player’s strengths and weaknesses and the ability to adapt and re-adapt to him more quickly than he can do the same are skills from which a successful poker player derives his edge.
Whereas Chen and Ankenman advocate playing optimally against unknown opponents, I would argue that you often can and should assume and default to exploiting certain weaknesses until you see some evidence to the contrary. Balancing one’s river betting range in order to avoid exploitation by a check-raise bluff, for instance, is a poor default strategy because very few poker players are capable of such a tactic.
The real strength of The Mathematics of Poker, in my opinion, is not that it will help you to play a near-optimal strategy. Rather, it will help you to understand optimal strategy so that you can better recognize and exploit your opponents’ inevitable deviations from it. For example, one toy game illustrates how a player in position must value bet and bluff fewer hands when his opponent is allowed to check-raise than in a game where he is not. The lesson I take from this is that against an opponent who rarely check-raises the river, I should value bet and bluff more often than optimal strategy would suggest.
But ultimately, these are shortcomings, not flaws. The mathematics are there to illustrate the game theory that underlies poker. Even with the supplemental explanations and synopses, The Mathematics of Poker is a demanding read. It asks a lot of the reader both in following the arguments and in making the jump from toy games to real life poker. Those who invest the requisite time and energy, however, will be rewarded with a deeper understanding of how to exploit their opponents and how to avoid such exploitation themselves.
If you found this review helpful and choose to purchase this book, please use this affiliate link to do so: The Mathematics of Poker